Doğrusal Cebir II: Kare Matrisler, Hesaplama Yöntemleri ve Uygulamalar / Linear Algebra II: Square Matrices, Calculation Methods and Applications from Coursera

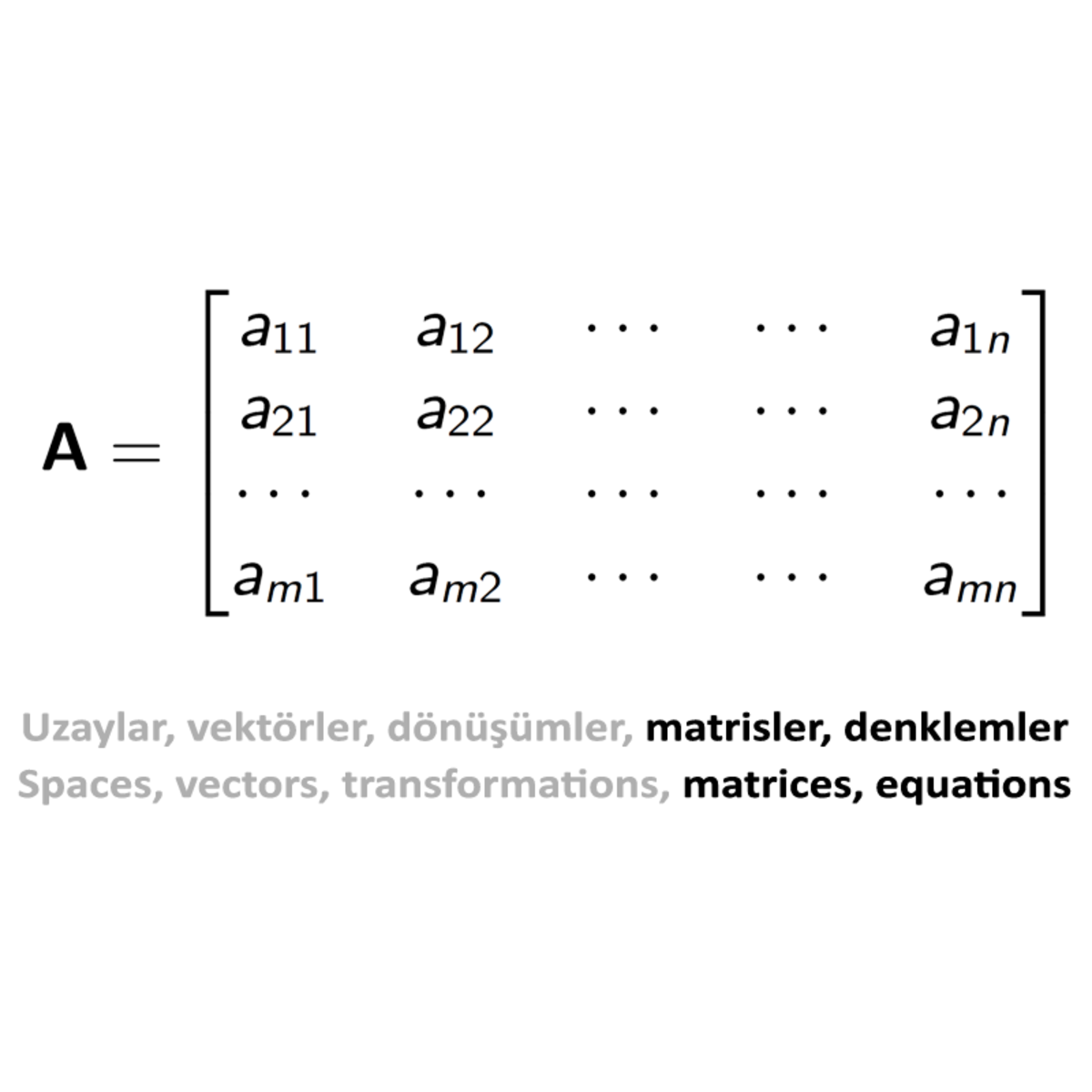
Doğrusal cebir ikili dizinin ikincisi olan bu ders birinci derste verilen temel bilgilerin üzerine eklemeler yapılarak tamamen matris işlemleri ve uygulamalarını kapsamaktadır. Cebirsel denklem sistemleri, sonuçların tekilliği ve var olup olmadığı, determinantlar ve onların doğal olarak nasıl oluştuğu, öz değer problemleri ve onların matris fonksiyonlarına uygulanışı vb. konulara derste değinilmektedir. Ders gerçek yaşamdan gelen uygulamaları da tanıtmaya önem veren “içerikli yaklaşımla” tasarlanmıştır.

Bölümler:

Bölüm 1: Doğrusal Cebir I'in Özeti

Bölüm 2: Kare Matrislerde Determinant

Bölüm 3: Kare Matrislerin Tersi

Bölüm 4: Kare Matrislerde Özdeğer Sorunu

Bölüm 5: Matrislerin Köşegenleştirilmesi

Bölüm 6: Matris Fonksiyonları

Bölüm 7: Matrislerle Diferansiyel Denklem Takımları

-----------

This second of the sequence of two courses builds on the fundamentals of the first course, is entirely on matrix algebra and applications. Specifically, the studies include systems of algebraic equations including the existence and uniqueness of solutions, determinants and how they arise naturally, eigenvalue problems with their applications to diagonalization and matrix functions. The course is designed in the same spirit as the first one with a “content based” emphasis, answering the “why” and “where“ of the topics, as much as the traditional “what” and “how” leading to “definitions” and “proofs”.

Chapters:

Chapter 1: Summary of Linear Algebra I

Chapter 2: Determinant

Chapter 3: Inverse of Square Matrices

Chapter 4: Eigenvalue Problem in Square Matrices

Chapter 5: Diagonalization of Matrices

Chapter 6: Matrix Functions

Chapter 7: Matrices and Systems of Differential Equations

-----------

Kaynak: Attila Aşkar, “Doğrusal cebir”. Bu kitap dört ciltlik dizinin üçüncü cildidir. Dizinin diğer kitapları Cilt 1 “Tek değişkenli fonksiyonlarda türev ve entegral”, Cilt 2: "Çok değişkenli fonksiyonlarda türev ve entegral" ve Cilt 4: “Diferansiyel denklemler” dir.

Source: Attila Aşkar, Linear Algebra, Volume 3 of the set of Vol1: Calculus of Single Variable Functions, Volume 2: Calculus of Multivariable Functions and Volume 4: Differential Equations.

What's inside

Syllabus

Doğrusal Cebir I'in Özeti / Summary of Linear Algebra I

Kare Matrislerde Determinant / Determinant in Square Matrices

Kare Matrislerin Tersi / Inverse of Square Matrices

Kare Matrislerde Özdeğer Sorunu / Eigenvalue Problem in Square Matrices

Matrislerin Köşegenleştirilmesi / Diagonalization of Matrices

Matris Fonksiyonları / Matrix Functions

Matrislerle Diferansiyel Denklem Takımları / Matrices and Systems of Differential Equations

Good to know

Know what's good

, what to watch for

, and possible dealbreakers

Aynı kurum tarafından verilen, aynı konu hakkındaki benzer isimli eğitim dizisinin bir parçasıdır

Teorik ve pratik uygulamalar içerir

Konuların 'neden' ve 'nerede' sorularını cevaplayarak geleneksel 'ne' ve 'nasıl' sorularına ek olarak öğretir

İkinci derste olduğundan, Doğrusal Cebir I'deki temel bilgileri ön koşul olarak gerektirir

Ders uygulamaları, gerçek hayattan örnekler üzerinden tasarlanmıştır

Save this course

Save Doğrusal Cebir II: Kare Matrisler, Hesaplama Yöntemleri ve Uygulamalar / Linear Algebra II: Square Matrices, Calculation Methods and Applications to your list so you can find it easily later:

Save

Reviews summary

Course appreciated

The Doğrusal Cebir II course gives learners an in-depth look at matrices through examples and applications in real life. Reviews indicate that instructors teach concepts well.

Course has content for current educational needs.

"Konu benim su anki egitimin icin cok yararli..."

Course shows examples from real situations.

Instructors explain concepts well.

"Konu benim su anki egitimin icin cok yararli ve hoca tarafindan gayet iyi anlatilmis."

Questions are modeled well but too numerous.

"No. of questions can be reduced since it is of same model."

Activities

Be better prepared before your course. Deepen your understanding during and after it. Supplement your coursework and achieve mastery of the topics covered in Doğrusal Cebir II: Kare Matrisler, Hesaplama Yöntemleri ve Uygulamalar / Linear Algebra II: Square Matrices, Calculation Methods and Applications with these activities:

Review Linear Algebra I

Show steps

Revisit the key concepts covered in Linear Algebra I will help you build a strong foundation for this course.

Browse courses on Linear Algebra

Show steps

Read through your notes and textbooks from Linear Algebra I.
Solve practice problems and review examples.
Take a practice test or quiz to assess your understanding.

Develop a Visual Study Guide

Show steps

Creating a visual study guide will help you organize and reinforce your understanding of matrices and determinants.

Browse courses on Matrices

Show steps

Gather key concepts, definitions, and formulas related to matrices and determinants.
Use diagrams, flowcharts, or mind maps to represent the relationships between these concepts.
Add examples and explanations to illustrate the concepts.

Explore Matrix Operations in MATLAB

Show steps

Hands-on practice with matrix operations in MATLAB will complement the theoretical concepts covered in the course.

Browse courses on Matrix Operations

Show steps

Find online tutorials or courses on MATLAB.
Follow the tutorials and practice writing MATLAB code for matrix operations.
Experiment with different matrix operations and explore their effects.

Two other activities

Expand to see all activities and additional details

Show all five activities

Solve Eigenvalue Problems

Show steps

Repeated practice in solving eigenvalue problems will enhance your proficiency in this critical topic.

Show steps

Find online resources or textbooks with eigenvalue problem exercises.
Solve as many problems as possible, focusing on different types of matrices.
Compare your solutions with provided answers or consult with the instructor for feedback.

Build a Matrix Calculator

Show steps

Developing a matrix calculator will provide practical experience in implementing matrix operations and deepen your understanding of their behavior.

Browse courses on Matrices

Show steps

Choose a programming language and environment.
Design the user interface and functionality of the calculator.
Implement matrix operations such as addition, subtraction, multiplication, and inversion.

Career center

Learners who complete Doğrusal Cebir II: Kare Matrisler, Hesaplama Yöntemleri ve Uygulamalar / Linear Algebra II: Square Matrices, Calculation Methods and Applications will develop knowledge and skills that may be useful to these careers:

Operations Research Analyst

Operations Research Analysts are responsible for using mathematical and analytical techniques to solve complex business problems. The course's focus on linear algebra is highly relevant, as linear programming is a fundamental tool in operations research. The course's coverage of matrix methods and differential equations is also very useful, as these techniques are used to model and analyze complex systems in a variety of industries.

See salaries and explore the career path for Operations Research Analyst

Actuary

Actuaries are responsible for assessing and managing financial risk. The course's focus on linear algebra is highly relevant, as matrix algebra is used extensively in actuarial science to model complex financial systems and make predictions about future events. The course's coverage of determinants and eigenvalues is also relevant, as these concepts are used in risk management and insurance pricing.

See salaries and explore the career path for Actuary

Quantitative Analyst

Quantitative Analysts are responsible for developing and using mathematical and statistical models to analyze financial data and make investment decisions. The course's focus on linear algebra is highly relevant, as matrix algebra is used extensively in quantitative finance to model complex financial systems and make predictions about future market behavior. The course's coverage of determinants and eigenvalues is also relevant, as these concepts are used in portfolio optimization and risk management.

See salaries and explore the career path for Quantitative Analyst

Data Analyst

Data Analysts are responsible for collecting, cleaning, and analyzing data to help businesses make better decisions. The course's focus on linear algebra and matrix functions is very relevant to this role. Eigenvalues and eigenvectors are essential concepts in many data mining techniques and are used to identify patterns and relationships in large datasets. The concepts of vectors, matrices, and transformations are also fundamental to statistical modeling, which is another integral part of a Data Analyst's role.

See salaries and explore the career path for Data Analyst

Physicist

Physicists are responsible for studying the laws of nature and developing theories to explain physical phenomena. The course's focus on linear algebra is highly relevant to this role, as linear algebra is used extensively in physics to describe and predict the behavior of physical systems. The course's coverage of determinants and eigenvalues is also relevant, as these concepts are used in quantum mechanics and other areas of physics.

See salaries and explore the career path for Physicist

Computer Scientist

Computer Scientists are responsible for designing and developing computer systems and applications. The course's focus on linear algebra is highly relevant, as linear algebra is used extensively in computer science to model complex systems and solve computational problems. The course's coverage of determinants and eigenvalues is also relevant, as these concepts are used in computer graphics and machine learning.

See salaries and explore the career path for Computer Scientist

Software Engineer

Software Engineers are responsible for designing, developing, and maintaining software systems. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of software engineering, such as computer graphics and machine learning. The course's coverage of determinants and eigenvalues is also moderately relevant, as these concepts are used in some software engineering applications.

See salaries and explore the career path for Software Engineer

Aerospace Engineer

Aerospace Engineers are responsible for designing, developing, and maintaining aircraft and spacecraft. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of aerospace engineering, such as flight dynamics and control systems. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the motion of aircraft and spacecraft.

See salaries and explore the career path for Aerospace Engineer

Mechanical Engineer

Mechanical Engineers are responsible for designing, developing, and maintaining mechanical systems. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of mechanical engineering, such as structural analysis and vibration analysis. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the behavior of mechanical systems.

See salaries and explore the career path for Mechanical Engineer

Electrical Engineer

Electrical Engineers are responsible for designing, developing, and maintaining electrical systems. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of electrical engineering, such as circuit analysis and signal processing. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the behavior of electrical systems.

See salaries and explore the career path for Electrical Engineer

Civil Engineer

Civil Engineers are responsible for designing, developing, and maintaining civil infrastructure, such as bridges, buildings, and roads. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of civil engineering, such as structural analysis and geotechnical engineering. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the behavior of civil structures.

See salaries and explore the career path for Civil Engineer

Chemical Engineer

Chemical Engineers are responsible for designing, developing, and maintaining chemical processes. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of chemical engineering, such as process modeling and control. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the behavior of chemical processes.

See salaries and explore the career path for Chemical Engineer

Biomedical Engineer

Biomedical Engineers are responsible for designing, developing, and maintaining biomedical devices and systems. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of biomedical engineering, such as medical imaging and signal processing. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the behavior of biological systems.

See salaries and explore the career path for Biomedical Engineer

Materials Scientist

Materials Scientists are responsible for studying the properties and behavior of materials. The course's focus on linear algebra is moderately relevant to this role, as linear algebra is used in some areas of materials science, such as crystallography and electronic structure calculations. The course's coverage of differential equations is also moderately relevant, as these equations are used to model the behavior of materials under different conditions.

See salaries and explore the career path for Materials Scientist

Research Scientist

Research Scientists are responsible for the scientific design of experiments and then carry out those experiments in a detail-oriented manner to ensure accurate results. They are also responsible for documenting their experiments and research findings. Many jobs at this level are heavily quantitative in orientation. This course, with its emphasis on linear algebra and matrix functions, may be useful for preparing to work with differential equations, which are ubiquitous in scientific research. The in-depth coverage of matrix diagonalization and matrix functions also make this course relevant to a research career.

See salaries and explore the career path for Research Scientist