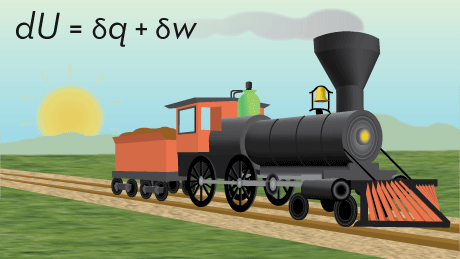
This introductory physical chemistry course examines the connections between molecular properties and the behavior of macroscopic chemical systems.
What's inside
Syllabus
Module 1
This module includes philosophical observations on why it's valuable to have a broadly disseminated appreciation of thermodynamics, as well as some drive-by examples of thermodynamics in action, with the intent being to illustrate up front the practical utility of the science, and to provide students with an idea of precisely what they will indeed be able to do themselves upon completion of the course materials (e.g., predictions of pressure changes, temperature changes, and directions of spontaneous reactions). The other primary goal for this week is to summarize the quantized levels available to atoms and molecules in which energy can be stored. For those who have previously taken a course in elementary quantum mechanics, this will be a review. For others, there will be no requirement to follow precisely how the energy levels are derived--simply learning the final results that derive from quantum mechanics will inform our progress moving forward. Homework problems will provide you the opportunity to demonstrate mastery in the application of the above concepts.
Read more
Syllabus
Good to know
Save this course
Reviews summary
Highly-rated molecular thermodynamics course
Activities
Read Atkins' Inorganic Chemistry
Show steps
Atkins' Inorganic Chemistry is a classic textbook that provides a comprehensive overview of inorganic chemistry. This book will help you to understand the basic principles of thermodynamics and how they apply to inorganic systems.
View
Physical Chemistry for the Life Sciences
on Amazon
Show steps
-
Read through the chapters on thermodynamics.
-
Take notes on the key concepts.
-
Do the practice problems at the end of each chapter.
Review Chemical Equations
Show steps
Reviewing chemical equations and stoichiometry will help you understand the language of chemistry and how to interpret and manipulate chemical reactions. This will give you a strong foundation for the rest of the course.
Show steps
-
Read through your notes or textbook on chemical equations and stoichiometry.
-
Practice balancing chemical equations.
-
Do some practice problems on stoichiometry.
Organize Your Notes
Show steps
Organizing your notes will help you to stay on top of the material and to be better prepared for exams.
Show steps
-
Go through your notes and make sure that they are complete.
-
Organize your notes into a logical order.
-
Create a system for storing and retrieving your notes.
Five other activities
Expand to see all activities and additional details
Show all eight activities
Join a Study Group
Show steps
Joining a study group will allow you to discuss the course material with other students and get help with difficult concepts. This can be a great way to improve your understanding of the material and to prepare for exams.
Show steps
-
Find a study group that meets regularly.
-
Attend the study group meetings and participate in the discussions.
-
Help other students with their questions.
Solve Thermodynamics Problems
Show steps
Solving practice problems will help you develop your problem-solving skills and apply the concepts of thermodynamics to real-world situations.
Browse courses on
Thermodynamics
Show steps
-
Find a set of practice problems on thermodynamics.
-
Solve the problems.
-
Check your answers against the answer key.
Learn about Molecular Partition Functions
Show steps
Molecular partition functions are a key concept in thermodynamics and statistical mechanics. By understanding how to calculate and interpret partition functions, you will gain a deeper understanding of the behavior of molecules and their interactions.
Show steps
-
Find a tutorial on molecular partition functions.
-
Watch the tutorial and take notes.
-
Try to apply the concepts you learned to some practice problems.
Build a Thermodynamics Calculator
Show steps
Building a thermodynamics calculator will help you to apply your knowledge of thermodynamics to a practical problem. This will help you to see how thermodynamics can be used to solve real-world problems.
Browse courses on
Thermodynamics
Show steps
-
Choose a programming language and development environment.
-
Design the interface of your calculator.
-
Write the code for your calculator.
-
Test your calculator against known results.
Develop a Thermodynamics Model
Show steps
Developing a thermodynamics model will allow you to apply the concepts of thermodynamics to a real-world problem. This will help you to see how thermodynamics can be used to solve practical problems.
Browse courses on
Thermodynamics
Show steps
-
Identify a problem that can be solved using thermodynamics.
-
Develop a mathematical model of the problem.
-
Use the model to make predictions about the behavior of the system.
-
Test the model against experimental data.
Read Atkins' Inorganic Chemistry
Show steps
Atkins' Inorganic Chemistry is a classic textbook that provides a comprehensive overview of inorganic chemistry. This book will help you to understand the basic principles of thermodynamics and how they apply to inorganic systems.
View
Physical Chemistry for the Life Sciences
on Amazon
Show steps
- Read through the chapters on thermodynamics.
- Take notes on the key concepts.
- Do the practice problems at the end of each chapter.
Review Chemical Equations
Show steps
Reviewing chemical equations and stoichiometry will help you understand the language of chemistry and how to interpret and manipulate chemical reactions. This will give you a strong foundation for the rest of the course.
Show steps
- Read through your notes or textbook on chemical equations and stoichiometry.
- Practice balancing chemical equations.
- Do some practice problems on stoichiometry.
Organize Your Notes
Show steps
Organizing your notes will help you to stay on top of the material and to be better prepared for exams.
Show steps
- Go through your notes and make sure that they are complete.
- Organize your notes into a logical order.
- Create a system for storing and retrieving your notes.
Join a Study Group
Show steps
Joining a study group will allow you to discuss the course material with other students and get help with difficult concepts. This can be a great way to improve your understanding of the material and to prepare for exams.
Show steps
- Find a study group that meets regularly.
- Attend the study group meetings and participate in the discussions.
- Help other students with their questions.
Solve Thermodynamics Problems
Show steps
Solving practice problems will help you develop your problem-solving skills and apply the concepts of thermodynamics to real-world situations.
Browse courses on
Thermodynamics
Show steps
- Find a set of practice problems on thermodynamics.
- Solve the problems.
- Check your answers against the answer key.
Learn about Molecular Partition Functions
Show steps
Molecular partition functions are a key concept in thermodynamics and statistical mechanics. By understanding how to calculate and interpret partition functions, you will gain a deeper understanding of the behavior of molecules and their interactions.
Show steps
- Find a tutorial on molecular partition functions.
- Watch the tutorial and take notes.
- Try to apply the concepts you learned to some practice problems.
Build a Thermodynamics Calculator
Show steps
Building a thermodynamics calculator will help you to apply your knowledge of thermodynamics to a practical problem. This will help you to see how thermodynamics can be used to solve real-world problems.
Browse courses on
Thermodynamics
Show steps
- Choose a programming language and development environment.
- Design the interface of your calculator.
- Write the code for your calculator.
- Test your calculator against known results.
Develop a Thermodynamics Model
Show steps
Developing a thermodynamics model will allow you to apply the concepts of thermodynamics to a real-world problem. This will help you to see how thermodynamics can be used to solve practical problems.
Browse courses on
Thermodynamics
Show steps
- Identify a problem that can be solved using thermodynamics.
- Develop a mathematical model of the problem.
- Use the model to make predictions about the behavior of the system.
- Test the model against experimental data.
Career center
Physical Chemist
Theoretical Chemist
Computational Chemist
Quantum Chemist
Chemical Engineer
Materials Scientist
Polymer Scientist
Nanotechnologist
Biochemist
Pharmaceutical Scientist
Environmental Scientist
Technical Writer
Teacher
Patent Attorney
Science Writer
Reading list
Share
Similar courses
OpenCourser helps millions of learners each year. People visit us to learn workspace skills, ace their exams, and nurture their curiosity.
Our extensive catalog contains over 50,000 courses and twice as many books. Browse by search, by topic, or even by career interests. We'll match you to the right resources quickly.
Find this site helpful? Tell a friend about us.
We're supported by our community of learners. When you purchase or subscribe to courses and programs or purchase books, we may earn a commission from our partners.
Your purchases help us maintain our catalog and keep our servers humming without ads.
Thank you for supporting OpenCourser.


