Sets
An Introduction to Sets
Sets are a fundamental concept in mathematics, forming the bedrock upon which many other mathematical ideas are built. At its most basic level, a set is simply a collection of distinct objects, considered as an object in its own right. These objects, called elements or members of the set, can be anything: numbers, people, letters of the alphabet, other sets, and so on. The defining characteristic is that a set is determined entirely by its members, regardless of order or repetition.
Understanding sets unlocks pathways into diverse fields, from pure mathematics and logic to computer science, linguistics, and economics. The elegance of set theory lies in its ability to formalize the intuitive idea of grouping things together, providing a precise language for complex relationships. Exploring sets can be intellectually rewarding, offering insights into the structure of information and the nature of mathematical reasoning itself. It's a concept that appears simple on the surface but reveals profound depth upon closer examination.
Introduction to Sets
What is a Set? Defining the Basics
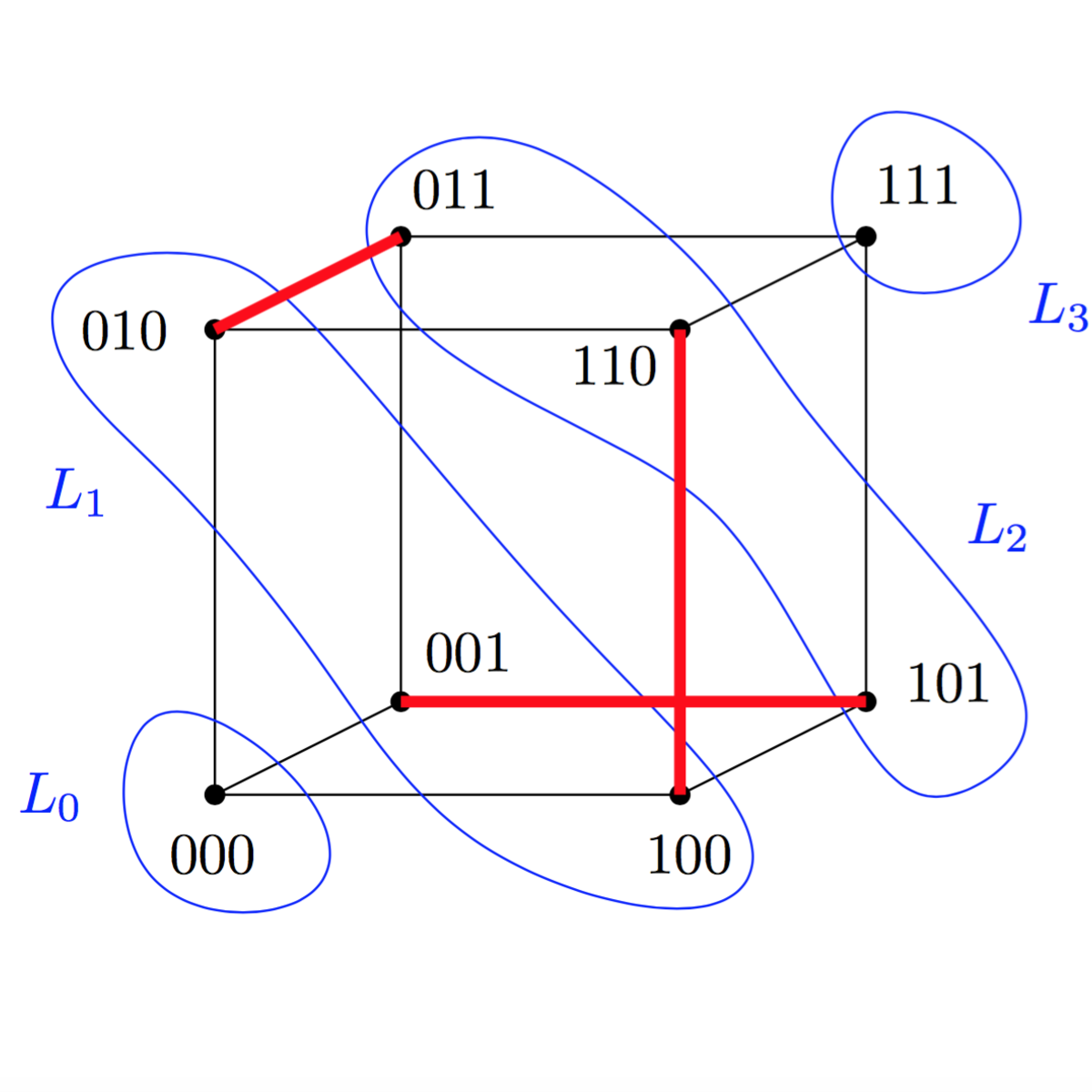
Imagine you have a box where you keep your favorite marbles. That box represents a set, and each unique marble inside is an element of that set. In mathematics, a set is formally defined as a well-defined collection of distinct objects. "Well-defined" means that given any object, we can definitively say whether it belongs to the set or not. "Distinct" means that each object appears only once; duplicates are ignored. For example, the set of vowels in the English alphabet is {a, e, i, o, u}. The order doesn't matter, so {u, o, i, e, a} represents the same set. Similarly, if we listed 'a' twice, it would still be the same set, containing just one 'a'.
Sets are typically denoted using curly braces { }. For instance, the set containing the numbers 1, 2, and 3 is written as {1, 2, 3}. We use the symbol ∈ to indicate that an element belongs to a set (e.g., 2 ∈ {1, 2, 3}) and ∉ to indicate that it does not (e.g., 4 ∉ {1, 2, 3}). An important concept is the empty set, denoted by {} or ∅, which is the unique set containing no elements at all. Think of it as an empty box – it's still a box (a set), just one without anything inside.
Another key idea is that of a subset. Set A is a subset of set B if every element of A is also an element of B. This is denoted by A ⊆ B. For example, {1, 2} is a subset of {1, 2, 3}. Every set is considered a subset of itself, and the empty set is a subset of every set. These basic definitions and notations form the language of set theory, allowing us to express complex ideas about collections and their relationships with clarity and precision. Understanding these fundamentals is the first step towards exploring the richer landscape of mathematics and its applications.
These introductory courses provide a solid grounding in the mathematical language and concepts needed to understand sets.
A Brief History of Set Theory
While the idea of collecting objects is ancient, the formal mathematical study of sets began relatively recently, primarily in the latter half of the 19th century. German mathematician Georg Cantor is widely regarded as the founder of set theory. Between 1874 and 1897, Cantor published a series of papers that introduced the core concepts, including the idea of different sizes of infinity (cardinality) and the rigorous treatment of infinite sets.
Cantor's work was revolutionary and initially met with resistance from some prominent mathematicians of his time who were uncomfortable with the concept of actual infinity. However, the power and utility of his ideas soon became apparent. Set theory provided a potential foundation for all of mathematics, offering a way to define concepts like numbers, functions, and relations in terms of sets. Figures like Richard Dedekind and later Bertrand Russell and Ernst Zermelo contributed significantly to its development and axiomatization.
The discovery of paradoxes, such as Russell's paradox (consider the set of all sets that do not contain themselves), highlighted the need for a more careful, axiomatic foundation. This led to the development of axiomatic set theories, like Zermelo-Fraenkel set theory (ZF) and ZFC (ZF with the Axiom of Choice), which provide rigorous frameworks to avoid such contradictions. Today, set theory, primarily in the form of ZFC, serves as the standard foundation for most modern mathematics.
These books delve into the historical context and foundational aspects of set theory.
Why Sets Matter: Importance Across Disciplines
Set theory is far more than an abstract mathematical curiosity; it is a foundational language that underpins numerous areas of mathematics and extends its influence into many applied fields. In pure mathematics, nearly every structure—from groups and rings in algebra to topological spaces and measure spaces in analysis—is defined in terms of sets and operations on them. It provides the basic vocabulary and framework for mathematical proof and reasoning.
Beyond pure mathematics, sets are indispensable in computer science. They form the basis for understanding data structures (like hash sets and trees), database theory (relational algebra is heavily based on set operations), algorithm design, and formal language theory. The ability to define, manipulate, and reason about collections of data is central to programming and software development.
Furthermore, concepts from set theory find applications in fields like linguistics (analyzing grammatical structures), economics (modeling preferences and choices), philosophy (exploring logic and ontology), and statistics (defining probability spaces and events). The clarity and rigor provided by set theory allow for precise modeling and analysis in these diverse domains. Mastering sets provides a versatile tool applicable across a wide spectrum of intellectual and practical pursuits.
Mathematical Foundations of Sets
Working with Sets: Operations
Just as we perform arithmetic operations like addition and multiplication on numbers, we can perform operations on sets to create new sets from existing ones. The three most fundamental operations are union, intersection, and complement. The union of two sets A and B, denoted A ∪ B, is the set containing all elements that are in A, or in B, or in both. For example, if A = {1, 2, 3} and B = {3, 4, 5}, then A ∪ B = {1, 2, 3, 4, 5}. Think of it as combining the contents of both sets into one, removing duplicates.
The intersection of two sets A and B, denoted A ∩ B, is the set containing all elements that are common to both A and B. Using the same sets A and B above, A ∩ B = {3}, because 3 is the only element present in both sets. If two sets have no elements in common, their intersection is the empty set (∅), and the sets are called disjoint.
The complement of a set A, often denoted A' or Aᶜ, refers to the elements not in A. This operation requires defining a universal set (U), which contains all possible elements under consideration in a particular context. The complement of A (relative to U) is the set of all elements in U that are not in A. For example, if U = {1, 2, 3, 4, 5} and A = {1, 2, 3}, then A' = {4, 5}. Another related concept is the set difference, A B or A - B, which is the set of elements in A but not in B. For our example sets, A B = {1, 2}. These operations allow us to precisely describe relationships and manipulate collections of objects.
These courses cover the fundamental operations and concepts within discrete mathematics, where set theory plays a central role.
Counting the Uncountable: Cardinality and Infinite Sets
Cardinality refers to the "size" of a set, or the number of elements it contains. For finite sets, this is straightforward: the set {a, b, c} has a cardinality of 3. We denote the cardinality of a set A as |A|. Things become much more interesting, and perhaps counterintuitive, when dealing with infinite sets. Georg Cantor's groundbreaking work showed that not all infinite sets have the same size; there are different "levels" of infinity.
Two sets are said to have the same cardinality if there exists a one-to-one correspondence (a bijection) between their elements. This means we can pair up every element in the first set with exactly one element in the second set, with no elements left over in either set. Cantor demonstrated that the set of natural numbers (ℕ = {1, 2, 3, ...}) has the same cardinality as the set of integers (ℤ = {..., -2, -1, 0, 1, 2, ...}) and even the set of rational numbers (ℚ, fractions). Sets with the same cardinality as ℕ are called countably infinite.
Perhaps more surprisingly, Cantor proved that the set of real numbers (ℝ, including all rational and irrational numbers) has a larger cardinality than the set of natural numbers. This means it's impossible to create a one-to-one correspondence between ℕ and ℝ; the real numbers are "more numerous" than the natural numbers. Such sets are called uncountably infinite. This discovery opened up a whole new realm of mathematics dealing with the properties and hierarchy of infinite sets, a topic central to advanced set theory and foundational studies.
Exploring the nuances of cardinality and infinity requires a deeper dive into set theory's formal aspects.
The Logic Connection: Sets and Proofs
Set theory is deeply intertwined with mathematical logic and proof techniques. The language of sets provides a framework for expressing logical statements precisely. For instance, the statement "All dogs are mammals" can be translated into set theory as "The set of dogs is a subset of the set of mammals." Logical operators like AND (∧), OR (∨), and NOT (¬) have direct parallels in set operations: intersection (∩), union (∪), and complement ('), respectively.
Understanding set theory is crucial for constructing rigorous mathematical proofs. Many proofs involve demonstrating relationships between sets, such as proving that one set is a subset of another (A ⊆ B) or that two sets are equal (A = B). A common technique to prove A = B is to show double inclusion: first prove A ⊆ B, and then prove B ⊆ A. This involves taking an arbitrary element from the first set and showing, through logical deduction, that it must also belong to the second set, and vice versa.
Venn diagrams, while often used for illustration, are informal tools. Formal proofs rely on the definitions of sets and their operations, combined with logical rules of inference. Techniques like proof by contradiction are also common; for example, to prove A ∩ B = ∅, one might assume there exists an element x in the intersection and derive a contradiction. Developing proficiency in writing proofs involving sets builds essential skills in logical reasoning applicable throughout mathematics and related fields.
This course focuses specifically on developing proof-writing skills within the context of set theory.
These books explore the connections between set theory, logic, and mathematical reasoning.
Sets in Computer Science Applications
Organizing Data: Sets as Data Structures
In computer science, the abstract concept of a set manifests as concrete data structures used to store collections of unique items efficiently. Many programming languages provide built-in set implementations (like Set in Java, set in Python, std::set in C++). These structures guarantee that elements are unique and typically offer fast operations for adding elements, removing elements, and checking if an element is present in the set (membership testing).
The underlying implementation often uses hash tables (leading to hash sets) or balanced binary search trees (like red-black trees, leading to tree sets). Hash sets generally provide average-case constant time complexity (O(1)) for add, remove, and contains operations, making them extremely fast. Tree sets maintain elements in a sorted order and offer logarithmic time complexity (O(log n)) for these operations, which is useful when ordered traversal is needed.
Understanding the properties of mathematical sets informs the design and use of these data structures. Programmers leverage sets when they need to ensure uniqueness, perform quick lookups, or efficiently compute set operations like union, intersection, and difference on collections of data. Choosing the right set implementation depends on the specific requirements of the application, such as the need for ordering or the performance characteristics desired.
These courses cover data structures, including sets, within the context of specific programming languages.
Efficient Information Retrieval: Database Queries
Set theory forms the theoretical foundation for relational databases, which store data in tables (relations). Each row in a table can be thought of as an element in a set, and database query languages like SQL (Structured Query Language) heavily rely on set operations to retrieve and manipulate data.
For example, a SELECT DISTINCT query retrieves unique rows, effectively treating the result as a set. The UNION operator in SQL combines the results of two queries, analogous to set union (removing duplicate rows). INTERSECT finds common rows between two query results (set intersection), and EXCEPT (or MINUS in some SQL dialects) finds rows present in the first query result but not the second (set difference).
Database management systems (DBMS) use principles derived from set theory and relational algebra to optimize query execution. Understanding how these operations work allows database administrators and developers to write more efficient queries. Techniques like indexing are designed to speed up operations that essentially involve searching within sets (tables) or joining sets based on common attributes.
Algorithmic Thinking: Set-Based Patterns
Sets and set operations are powerful tools in algorithm design. Many problems can be simplified or solved more efficiently by framing them in terms of sets. For example, finding common elements between two lists can be efficiently solved by converting the lists to sets and performing a set intersection.
Algorithms for graph traversal, network analysis, and scheduling often utilize sets to keep track of visited nodes, available resources, or candidate solutions. Set-based operations provide concise ways to express complex conditions. For instance, checking if a path exists that covers a required set of nodes involves manipulating sets of visited locations.
Furthermore, probabilistic data structures like Bloom filters provide approximate set membership testing with very low memory usage, useful in large-scale systems where exact sets are too costly to store. Understanding set theory enables developers to recognize when a problem can be modeled using sets and to apply appropriate algorithms and data structures for optimal performance.
These courses touch upon algorithms and data structures where set concepts are applied.
Formal Education Pathways
Sets in Undergraduate Mathematics
Set theory is typically introduced early in an undergraduate mathematics curriculum, often within introductory courses on proofs, discrete mathematics, or foundational concepts. These initial encounters focus on basic definitions, notation, set operations (union, intersection, complement, power set), Venn diagrams, and the concept of cardinality for finite sets. The goal is to equip students with the fundamental language needed for higher-level mathematics courses.
As students progress, set-theoretic concepts become implicitly woven into various subjects. In abstract algebra, groups, rings, and fields are defined as sets with specific operations and axioms. In real analysis, topology is built upon open and closed sets. In probability theory, events are defined as subsets of a sample space. While dedicated courses purely on set theory might be elective, its principles are pervasive.
Courses specifically titled "Discrete Mathematics" often provide the most substantial undergraduate treatment of applied set theory, covering relations, functions (as special types of relations defined using sets), equivalence relations, partial orders, and combinatorics, all heavily reliant on set concepts. These courses are fundamental not only for math majors but also for computer science students, bridging the gap between mathematical foundations and computational applications.
This book covers foundational mathematics suitable for undergraduate study.
Advanced Study: Graduate Set Theory
At the graduate level, set theory transforms from a foundational tool into a distinct field of mathematical research. Courses delve into the axiomatic foundations, typically Zermelo-Fraenkel set theory with the Axiom of Choice (ZFC). Students explore the intricacies of infinite cardinals and ordinals, transfinite induction, and the construction of the number systems from set-theoretic axioms.
Advanced topics may include large cardinals, forcing (a powerful technique used to prove the independence of statements like the Continuum Hypothesis from ZFC), descriptive set theory (studying the structure of definable sets of real numbers), and inner model theory. These areas push the boundaries of mathematical logic and explore the limits of what can be known and proven within standard mathematical frameworks.
Graduate study in set theory requires a high level of abstract thinking and a strong background in mathematical logic and proofs. It appeals to students interested in the fundamental nature of mathematics, infinity, and the structure of mathematical truth. Research often involves constructing complex mathematical objects or proving the consistency or independence of various mathematical statements relative to the axioms of set theory.
These texts are often used in more advanced or graduate-level studies of set theory.
Research Frontiers in Foundations
Research in foundational mathematics, particularly set theory, continues to explore deep questions about the nature of mathematical objects and proof. Active areas include the study of large cardinals, which are hypothetical infinities with strong properties whose existence cannot be proven within ZFC but which have profound consequences throughout mathematics. Researchers investigate the consistency strength of various large cardinal axioms and their implications.
Forcing remains a central technique, used not only for independence proofs but also to construct intricate set-theoretic models with specific properties. Descriptive set theory continues to analyze the complexity of sets of real numbers and their connections to other areas like analysis and topology. Inner model theory seeks to build canonical models for set theory, potentially shedding light on questions like the Continuum Hypothesis.
These research areas often intersect with mathematical logic, model theory, and computability theory. Pursuing research in foundational mathematics requires dedication and a tolerance for highly abstract concepts. It offers the opportunity to contribute to our understanding of the very framework upon which mathematics is built.
Online Learning Strategies
Crafting Your Self-Study Curriculum
Learning set theory independently is achievable, especially with the wealth of online resources available. Start by identifying your goals: are you learning it as a foundation for other math subjects, for computer science applications, or out of pure interest? This will help you select appropriate materials. Begin with introductory resources that cover basic definitions, notation, and operations. Many university websites offer lecture notes for introductory discrete math or proofs courses.
Structure your learning progressively. Master the basics—definitions, subsets, union, intersection, complement, power sets—before moving to relations, functions, and cardinality. If your interest lies in computer science, focus on how these concepts relate to data structures and algorithms. If aiming for deeper mathematical understanding, explore infinite sets, ordinals, and cardinals. Use textbooks (like those listed elsewhere in this article) alongside online courses for a well-rounded perspective.
Platforms like OpenCourser aggregate courses from various providers, allowing you to compare syllabi and find options that fit your learning style and depth requirements. Create a schedule, set realistic milestones, and regularly review material. Don't just passively watch videos or read; actively work through examples and exercises to solidify your understanding. The OpenCourser Learner's Guide offers tips on structuring self-study and staying motivated.
These courses provide foundational mathematical knowledge, suitable for starting a self-study curriculum.
Learning by Doing: Project-Based Approaches
Abstract concepts like set theory become much clearer when applied. Incorporate project-based learning into your study plan. If you're learning programming alongside set theory, implement your own basic set data structure. Try building it using different underlying mechanisms like hash tables or linked lists, and compare their performance for operations like add, remove, and contains.
Another project could involve implementing algorithms that heavily rely on set operations. For example, write a program to find the union or intersection of large data files, treating each line as an element. Explore graph algorithms where sets are used to track visited nodes (like in Breadth-First Search or Depth-First Search) or components (like finding connected components).
Consider projects related to database concepts. You could simulate simple relational algebra operations (select, project, join, union, intersection, difference) using sets in your preferred programming language. Even small-scale projects like building a tool to analyze word frequencies in a text (using sets to store unique words) can reinforce understanding. Applying the theory makes it less abstract and highlights its practical utility.
These courses involve programming and data analysis, offering opportunities to apply set concepts in projects.
Checking Your Understanding: Assessment Methods
Regularly assessing your understanding is crucial when self-studying. Most online courses include quizzes and assignments that provide immediate feedback. Take these seriously, as they pinpoint areas needing review. Many standard textbooks also feature extensive exercise sets, often with solutions or hints for selected problems provided.
Beyond formal exercises, try explaining concepts in your own words. Can you clearly define a set, subset, union, and intersection? Can you explain the difference between countably and uncountably infinite sets? Teaching the material to someone else (even an imaginary audience) is an excellent way to test your grasp.
Look for online platforms or forums where you can discuss problems and solutions with peers. Engaging in discussions helps clarify doubts and exposes you to different perspectives. Consider tackling problems from online judges or competitive programming sites that involve set manipulation or set-based algorithms. Successfully solving these problems provides tangible proof of your applied understanding.
Industry Demand and Trends
Sets in Modern Computing: AI and Machine Learning
While perhaps less visible than calculus or linear algebra, set theory concepts remain relevant in advanced computing fields like Artificial Intelligence (AI) and Machine Learning (ML). Feature engineering, a critical step in building ML models, often involves selecting, combining, or transforming sets of input variables. Set operations can be used to manage and compare different feature sets during model development.
In areas like Natural Language Processing (NLP), sets are used to represent vocabularies or collections of relevant terms. Knowledge representation in AI sometimes utilizes set-theoretic structures to model relationships between concepts. Furthermore, the underlying theory of computation, which informs AI algorithm design, relies heavily on concepts rooted in set theory and formal languages.
While day-to-day work in AI/ML might focus more on specific algorithms and libraries, a solid grasp of foundational mathematics, including set theory, provides a deeper understanding of why certain methods work and enables more effective problem-solving and innovation. It contributes to the analytical rigor required to develop and evaluate complex AI systems.
Job Market Relevance
Direct job postings explicitly requiring "set theory expertise" are rare outside of academic research in foundational mathematics or theoretical computer science. However, the skills and concepts derived from studying set theory are highly valued implicitly across many technical roles, particularly in software development, data science, database administration, and algorithm design.
Employers seek candidates who can think logically, handle abstract concepts, and understand data structures and algorithms—all skills honed by studying set theory. Proficiency in programming languages often involves mastering their built-in collection types, including sets. Roles involving database design and query optimization benefit directly from an understanding of relational algebra's set-theoretic roots.
Therefore, while set theory itself isn't typically listed as a primary job requirement, it forms part of the essential mathematical and computational foundation expected in many technical careers. Demonstrating a strong grasp of discrete mathematics, data structures, and algorithms—areas heavily reliant on set theory—is key to succeeding in the job market for these roles. According to industry analyses, demand for professionals with strong analytical and computational skills remains robust across various sectors.
Transferability Across Industries
The logical reasoning and structured thinking cultivated through learning set theory are highly transferable skills applicable across numerous industries. The ability to precisely define categories, understand relationships between groups, and manipulate collections of information is valuable in fields beyond technology.
In finance, set concepts can inform portfolio construction or risk categorization. In logistics and operations research, sets can model resources, constraints, or locations. Market research and data analysis often involve segmenting populations or survey responses into distinct sets based on various criteria.
Even in less quantitative fields, the clarity of thought fostered by mathematical training, including set theory, is beneficial. The ability to break down complex problems, identify distinct components, and understand their interrelations is a core competency applicable to management, policy analysis, and strategic planning. While the specific terminology might differ, the underlying principles of classification and relationship mapping derived from set theory have broad applicability.
Ethical Considerations in Set Applications
Algorithmic Bias and Set Operations
When sets are used to categorize people or data points in algorithms, particularly in AI/ML systems, there's a risk of encoding or amplifying societal biases. For example, if a dataset used for training a hiring algorithm contains historical biases, defining sets based on certain attributes (e.g., "qualified candidates," "high-risk applicants") might inadvertently perpetuate discrimination.
Set operations themselves are neutral, but their application to biased data or the choice of criteria for set membership can lead to unfair outcomes. An intersection operation might disproportionately exclude individuals belonging to multiple minority groups. Defining complements (e.g., "not qualified") based on flawed criteria can unfairly penalize certain demographics.
Addressing this requires careful consideration of how sets are defined and used within algorithms. It involves auditing data for biases, scrutinizing the criteria for set membership, and evaluating the fairness of outcomes across different demographic groups. Ethical algorithm design necessitates awareness of how seemingly objective mathematical operations can interact with biased data to produce inequitable results.
Data Privacy Concerns
Set operations can inadvertently reveal sensitive information, particularly when dealing with large datasets containing personal data. Techniques like set intersection, used to find common elements between datasets, can potentially de-anonymize individuals if not handled carefully.
For example, intersecting a supposedly anonymized dataset of medical conditions with another dataset containing zip codes and ages might allow for the re-identification of individuals. Even aggregate information about set sizes or overlaps can sometimes leak private information through inference attacks.
Protecting privacy in set-based computations is an active area of research, leading to techniques like differential privacy, which adds controlled noise to computations to protect individual data while still allowing for meaningful aggregate analysis. Secure multi-party computation allows different parties to compute functions over their combined datasets (like set intersection size) without revealing their individual data to each other. Implementing systems that use set operations on sensitive data requires robust privacy-preserving techniques.
Regulation and Mathematical Systems
As algorithms and data-driven systems become more influential in society, questions arise about how to regulate their use, particularly when they involve complex mathematical concepts like set theory. Ensuring fairness, transparency, and accountability in systems that categorize and make decisions based on set memberships is a growing challenge.
Regulatory frameworks are evolving to address issues like algorithmic bias and data privacy. Regulations like the GDPR in Europe place restrictions on how personal data can be processed, impacting how set operations can be applied to user data. Discussions around AI ethics often touch upon the need for explainability – being able to understand why an algorithm placed an individual into a particular set or category.
Developing effective regulations requires collaboration between policymakers, technologists, and ethicists. It involves translating ethical principles into technical requirements and potentially establishing standards for how mathematical concepts are implemented in critical systems. The mathematical community has a role to play in explaining the capabilities and limitations of these techniques to inform responsible governance.
Challenges and Common Obstacles
Grasping Abstraction: Cognitive Hurdles
One of the primary challenges many learners face with set theory is its abstract nature. Moving from concrete examples (like a set of fruits) to abstract concepts (like the set of all functions from real numbers to real numbers, or infinite sets) requires a shift in thinking. Concepts like the empty set, subsets, and especially operations on infinite sets can feel counterintuitive at first.
Overcoming this requires patience and practice. Start with concrete examples and gradually work towards more abstract ones. Visual aids like Venn diagrams can be helpful initially, but it's crucial to eventually rely on the formal definitions. Working through many different types of problems helps build intuition for how abstract concepts behave.
Don't be discouraged if it doesn't click immediately. Abstract mathematical thinking is a skill that develops over time. Discussing concepts with peers or instructors, trying to explain them yourself, and revisiting definitions frequently can aid understanding. Recognizing that this abstraction is powerful—allowing broad applicability—can provide motivation.
Bridging Theory and Practice
Another common obstacle is connecting the formal theory of sets to its practical applications, particularly in computer science. Students might understand the definitions of union and intersection but struggle to see how they translate into efficient code or database queries. The notation and rigor of mathematical set theory can seem disconnected from the syntax of programming languages.
To bridge this gap, actively seek connections. When learning about a data structure like a hash set in programming, relate its properties (uniqueness, fast lookup) back to the mathematical definition of a set. When writing SQL queries, identify the underlying set operations (UNION, INTERSECT, EXCEPT). Implement set operations yourself in code to see the algorithms behind them.
Project-based learning, as discussed earlier, is particularly effective here. Building small applications that utilize set concepts forces you to translate theory into practice. Explore resources like Stack Overflow or programming tutorials that explicitly discuss the implementation of set-based algorithms or data structures.
Finding the Right Resources
Navigating the vast array of available learning resources can itself be a challenge. Some resources might be too theoretical, others too focused on specific programming implementations, and some might simply be unclear or inaccurate. Finding materials that match your learning style, background, and goals is key.
Start by looking for well-regarded textbooks or online courses with good reviews and clear prerequisites. University course websites often list recommended readings. Platforms like OpenCourser Browse help you explore and compare courses and books from different sources. Look for resources that provide plenty of examples and exercises with solutions.
Don't rely on a single resource. Combining a textbook with video lectures, online articles, and practical coding exercises provides a more comprehensive learning experience. If you get stuck on a concept in one resource, try looking it up in another – a different explanation might provide the necessary clarity. Building a personalized learning path often involves curating materials from multiple sources.
These books offer different perspectives and levels of detail on set theory, helping learners find a style that suits them.
International Perspectives
Global Approaches to Teaching Sets
The way set theory is introduced and emphasized in mathematics education can vary across different countries and educational systems. In some systems, a formal introduction to sets occurs early, even in primary or secondary school, as part of "New Math" initiatives (though these have seen varying degrees of success and persistence). In others, it's deferred until late secondary school or the beginning of university, typically within discrete mathematics or introductory logic courses.
Curricular emphasis also differs. Some systems focus heavily on the axiomatic foundations and connections to logic, reflecting a more theoretical approach. Others prioritize the practical applications in computer science and data structures. Cultural factors and pedagogical philosophies influence whether learning is more rote-based, discovery-oriented, or problem-driven.
Understanding these variations can be helpful for students moving between educational systems or collaborating internationally. It highlights that there isn't one single "correct" way to learn or teach foundational mathematics, and different approaches may emphasize different aspects or applications of set theory.
International Research Collaboration
Research in set theory and foundational mathematics is inherently international. Mathematicians from around the globe collaborate on problems, share results through journals and conferences, and visit institutions in different countries. Major centers for research in logic and set theory exist in North America, Europe, Israel, and other parts of the world.
Organizations like the Association for Symbolic Logic (ASL) foster international collaboration through publications and meetings. Online repositories like the arXiv preprint server allow researchers worldwide to share their work rapidly. This global interconnectedness accelerates progress and allows for diverse perspectives on challenging foundational questions.
For students considering research careers, awareness of the international landscape is important. Opportunities exist for study and research abroad, and engaging with the global community is essential for staying at the forefront of the field. The universal language of mathematics facilitates this cross-border collaboration.
Cultural Context in Mathematical Learning
While mathematical truths are universal, cultural context can influence how mathematics, including set theory, is perceived, taught, and learned. Language itself can shape understanding; the way concepts like infinity or collections are expressed might differ subtly across languages. Pedagogical traditions also vary, impacting student engagement and approaches to problem-solving.
Societal attitudes towards mathematics and abstract thinking can affect student motivation and persistence. Access to resources, quality of instruction, and emphasis on foundational subjects within national education priorities all play a role. Recognizing these contextual factors is important for developing effective and inclusive mathematics education globally.
Efforts to improve math education often involve international comparisons and sharing of best practices, acknowledging that cultural context matters while striving for universal understanding of fundamental concepts like sets. Initiatives promoting math literacy worldwide aim to equip individuals with the foundational reasoning skills needed in an increasingly complex world.
Frequently Asked Questions
What are the essential skills for careers involving set theory?
While direct "set theorist" roles are mainly academic, careers leveraging set theory concepts (software engineering, data science, database management) require a blend of skills. Strong logical reasoning and analytical thinking are paramount. Proficiency in discrete mathematics, including combinatorics and graph theory, is often necessary.
Specific technical skills include understanding data structures (especially hash tables, trees, sets, maps) and algorithms. Proficiency in relevant programming languages (like Python, Java, C++, SQL) is essential for implementation. For database roles, deep knowledge of relational algebra and SQL query optimization is key. Abstract thinking and the ability to model real-world problems using mathematical structures are crucial underlying competencies.
Furthermore, communication skills are important for explaining complex technical concepts to colleagues or stakeholders. Problem-solving aptitude and attention to detail are also highly valued. Essentially, careers related to set theory demand a combination of theoretical understanding, practical implementation skills, and general analytical abilities.
Can set theory be effectively self-taught?
Yes, set theory can be effectively self-taught, especially its foundational aspects and applications in computer science. Numerous high-quality resources are available, including online courses, textbooks, university lecture notes, and interactive websites. Success depends largely on the learner's discipline, motivation, and approach.
Key strategies for effective self-study include structuring your learning path, starting with the basics and progressing logically, actively working through exercises and problems (not just passively consuming material), seeking diverse resources to gain different perspectives, and regularly testing your understanding. Applying concepts through coding projects or practical examples significantly aids retention and comprehension.
While self-study is feasible, accessing feedback can be challenging. Participating in online forums, finding a study group, or utilizing online courses with instructor support or peer review can help overcome this. For very advanced or axiomatic set theory, the abstraction level increases significantly, and guidance from experienced mathematicians (through formal courses or mentorship) becomes more beneficial, though still not strictly impossible to learn independently with sufficient dedication.
Which industries have the highest demand for these skills?
The skills associated with understanding and applying set theory are in highest demand within the technology sector. Software development roles require knowledge of data structures (including sets) and algorithms. Data science and analytics positions rely on logical thinking and data manipulation skills, often involving set-like operations on datasets.
Database administration and design roles are heavily reliant on the principles of relational algebra, which is built on set theory. The fields of Artificial Intelligence and Machine Learning also require strong mathematical foundations, including discrete mathematics. Cybersecurity involves analyzing patterns and relationships, sometimes using set-based concepts.
Beyond tech, quantitative finance (quant roles) demands strong mathematical and logical reasoning. Research positions in academia (mathematics, computer science, philosophy, linguistics) may directly involve set theory. Essentially, any industry requiring rigorous data analysis, algorithm development, or logical system design will value the foundational skills honed by studying set theory.
What are the typical salary expectations?
Salary expectations vary widely based on the specific role, industry, geographic location, years of experience, and level of education. Since "set theory specialist" isn't a standard job title outside academia, salaries align with related roles like Software Engineer, Data Scientist, Database Administrator, or Research Scientist.
Entry-level software engineering roles in major tech hubs often offer competitive salaries, with significant potential for growth. Data science roles, particularly those requiring advanced degrees or specialized skills, also command high salaries. Database administrators typically have solid earning potential. Academic research positions have salaries determined by university and grant funding structures, which can vary significantly. You can research specific job titles on sites like the U.S. Bureau of Labor Statistics Occupational Outlook Handbook or commercial salary comparison websites for more precise, up-to-date figures relevant to your location and target role.
A strong foundation in mathematics and computer science, including concepts derived from set theory, generally correlates with higher earning potential in technical fields due to the demand for analytical and problem-solving skills.
What does career progression look like?
Career progression in fields related to set theory depends heavily on the chosen path (e.g., industry vs. academia). In software engineering, progression typically moves from junior developer to mid-level, senior, and then potentially to roles like tech lead, architect, or engineering manager. Specialization in areas like backend systems, databases, or algorithms can open specific career tracks.
In data science, one might progress from junior data scientist to senior data scientist, lead data scientist, or specialize in areas like machine learning engineering or data engineering management. Database administrators can advance to senior roles, database architects, or specialize in performance tuning or specific database technologies.
In academia, the path typically involves completing a Ph.D., postdoctoral research positions, and then seeking tenure-track faculty positions (Assistant, Associate, Full Professor). Progression involves research publications, teaching, securing grants, and service to the academic community. Each path requires continuous learning and adaptation to new technologies or research developments.
Are there continuing education requirements?
Formal continuing education requirements (like mandated credits) are uncommon in most industry roles related to set theory (e.g., software engineering, data science), unlike professions such as medicine or law. However, the practical need for continuous learning is extremely high due to the rapid evolution of technology, programming languages, tools, and methodologies.
Professionals in these fields must constantly update their skills to remain relevant and advance their careers. This often involves self-study, taking online courses, attending workshops or conferences, reading technical blogs and papers, and contributing to open-source projects. While not formally mandated, a commitment to lifelong learning is essential for long-term success.
In academia, continuous research and publication are inherent requirements for career progression. Staying current with the latest developments in set theory and related mathematical fields is crucial for faculty members. While not always framed as "continuing education," the expectation of ongoing scholarly activity serves a similar purpose.
Set theory, though abstract, provides a powerful lens for understanding structure and relationships in mathematics, computer science, and beyond. Whether pursued for academic depth or practical application, its principles offer valuable tools for logical thinking and problem-solving.