Determinants
An Introduction to Determinants
Determinants represent a fundamental concept within linear algebra, providing a single numerical value associated with every square matrix. This number encapsulates crucial information about the matrix and the linear transformation it represents. At a high level, the determinant helps us understand properties like invertibility (whether a system of equations represented by the matrix has a unique solution) and the geometric scaling effect of the transformation.
Exploring determinants offers a gateway into the elegant structures of linear algebra and their wide-ranging applications. Understanding how these numerical values arise and what they signify can unlock deeper insights into fields from engineering and physics to economics and computer science. For those mathematically inclined, the journey into determinants reveals intricate connections between algebra, geometry, and analysis, offering intellectually stimulating challenges and discoveries.
Grasping determinants is often a key step in mastering mathematics relevant to numerous technical and scientific careers. It forms part of the bedrock upon which advanced mathematical modeling and data analysis techniques are built.
Introduction to Determinants
What is a Determinant?
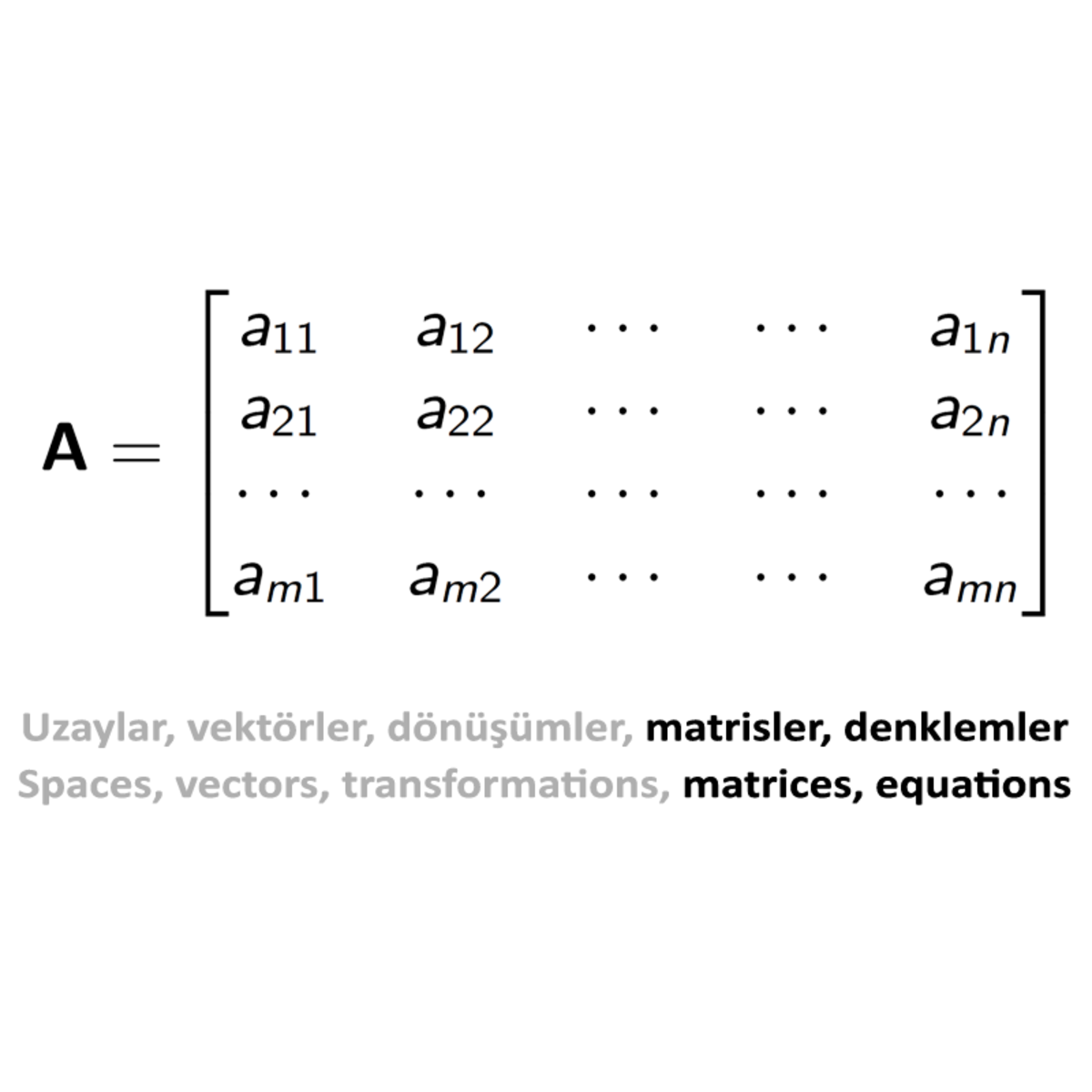
A determinant is a scalar value that can be computed from the elements of a square matrix (a matrix with the same number of rows and columns). For a 2x2 matrix, [[a, b], [c, d]], the determinant is calculated as ad - bc. For larger matrices, the calculation becomes more complex, often involving methods like cofactor expansion or row reduction.
This single number tells us significant things about the matrix. Perhaps most importantly, a non-zero determinant indicates that the matrix is invertible, meaning there exists another matrix (its inverse) that can "undo" the original matrix's transformation. This is directly linked to solving systems of linear equations: if the determinant of the coefficient matrix is non-zero, the system has a unique solution.
Geometrically, the absolute value of the determinant of a real matrix represents the scaling factor by which areas (in 2D), volumes (in 3D), or hypervolumes (in higher dimensions) are multiplied under the associated linear transformation. A determinant of 0 means the transformation collapses space onto a lower dimension (e.g., squashing a 3D object onto a plane or line), hence losing volume and becoming non-invertible.
These introductory courses can help build a foundational understanding of matrices and the concept of determinants.
For those seeking a standard textbook approach to begin their studies, these books offer comprehensive introductions.
Historical Context
The concept of determinants predates the formal definition of matrices. The idea emerged from the study of systems of linear equations. In the 3rd century BC, Chinese mathematicians used a method resembling Gaussian elimination to solve systems, implicitly touching upon ideas related to determinants. Later, in the 16th century, Gerolamo Cardano, while solving systems of two linear equations, provided a rule essentially equivalent to the determinant for a 2x2 matrix.
Significant development occurred in the late 17th and 18th centuries. Gottfried Wilhelm Leibniz, around 1693, and independently, Seki Kōwa in Japan, are credited with developing methods related to determinants for larger systems. Colin Maclaurin is also believed to have used determinants in his work published posthumously in 1748. Gabriel Cramer presented his rule for solving systems using determinants in 1750, although Maclaurin likely knew of it earlier.
The term "determinant" itself was introduced by Carl Friedrich Gauss in 1801 in his Disquisitiones Arithmeticae, though not in the modern sense; he used it for the discriminant of a quadratic form. Augustin-Louis Cauchy made substantial contributions in 1812, providing the first proof of the multiplication theorem (det(AB) = det(A)det(B)) and formalizing many properties. Arthur Cayley, in the mid-19th century, introduced the matrix notation that clarified and unified the theory, firmly establishing determinants within the broader context of matrix algebra.
Relevance in Linear Algebra
Determinants are a cornerstone concept in Linear Algebra. They serve as a criterion for matrix invertibility, which is fundamental for solving many problems. A square matrix A has an inverse if and only if its determinant, det(A), is non-zero. This property connects determinants directly to the existence and uniqueness of solutions to systems of linear equations of the form Ax = b.
Furthermore, determinants are intrinsically linked to eigenvalues and eigenvectors, another central topic in linear algebra. The eigenvalues of a matrix A are the roots of the characteristic polynomial, defined as det(A - λI) = 0, where λ represents the eigenvalues and I is the identity matrix. Eigenvalues and eigenvectors reveal deep structural information about the matrix and the transformation it represents.
Determinants also feature prominently in change of basis calculations and in defining the orientation and volume scaling of linear transformations. Their properties under row operations make them a useful tool in theoretical arguments and proofs within linear algebra and related fields.
These courses delve deeper into the role of determinants within the broader context of linear algebra.
Connection to Systems of Equations
One of the earliest motivations for studying determinants was their application to solving systems of linear equations. Consider a system of n linear equations with n unknowns, represented in matrix form as Ax = b, where A is the n x n coefficient matrix, x is the vector of unknowns, and b is the vector of constants.
Cramer's Rule, though often computationally inefficient for large systems compared to methods like Gaussian elimination, provides an explicit formula for the solution using determinants. It states that each unknown variable x_i can be found as the ratio of two determinants: the determinant of a matrix formed by replacing the i-th column of A with the vector b, divided by the determinant of A itself (provided det(A) is non-zero).
More fundamentally, the determinant of A tells us about the nature of the solutions. If det(A) ≠ 0, the system has a unique solution. If det(A) = 0, the system either has no solutions or infinitely many solutions, depending on the vector b. This connection highlights the power of determinants in analyzing the solvability and uniqueness of solutions for linear systems.
These resources explore the connection between matrix algebra, determinants, and solving systems of equations.
Mathematical Foundations
Linear Algebra Prerequisites
To fully appreciate determinants, a solid grounding in basic linear algebra concepts is necessary. This includes familiarity with vectors, vector spaces, matrix operations (addition, scalar multiplication, matrix multiplication), and the concept of linear independence. Understanding what a matrix represents, particularly as a linear transformation between vector spaces, is crucial.
Knowledge of different types of matrices, such as square matrices, identity matrices, diagonal matrices, and triangular matrices, is also helpful. Concepts like the rank of a matrix and the process of row reduction (Gaussian elimination) are closely related to determinant calculations and properties.
Essentially, determinants build upon the fundamental framework of linear algebra. While the calculation for a 2x2 matrix is simple, understanding its deeper meaning and generalizing it requires comfort with the language and tools of matrix theory.
These resources cover the essential linear algebra background needed before diving deep into determinants.
Key Properties and Theorems
Determinants possess several important properties that simplify calculations and are fundamental to theoretical developments. One key property is multiplicativity: for any two square matrices A and B of the same size, det(AB) = det(A)det(B). This property has far-reaching consequences in algebra and geometry.
Another crucial set of properties relates to row operations. Swapping two rows multiplies the determinant by -1. Multiplying a row by a scalar c multiplies the determinant by c. Adding a multiple of one row to another row does not change the determinant. These properties are the basis for computing determinants using row reduction to transform a matrix into an upper triangular form, where the determinant is simply the product of the diagonal entries.
Other important properties include: det(Aᵀ) = det(A), where Aᵀ is the transpose of A. If a matrix has a row or column of zeros, its determinant is zero. If a matrix has two identical rows or columns, its determinant is zero. The determinant of the identity matrix is 1. These properties, along with the definition (e.g., via cofactor expansion), form the core toolkit for working with determinants.
These more advanced resources delve into the properties and theorems governing determinants.
Computational Methods
Calculating determinants varies significantly with matrix size. For a 1x1 matrix [a], the determinant is just a. For a 2x2 matrix [[a, b], [c, d]], it's ad - bc. For a 3x3 matrix, the Rule of Sarrus provides a mnemonic: sum the products of the forward diagonals and subtract the products of the backward diagonals. However, this rule only works for 3x3 matrices.
For general n x n matrices, two primary methods exist: cofactor expansion and row reduction. Cofactor expansion (or Laplace expansion) expresses the determinant of an n x n matrix in terms of determinants of (n-1) x (n-1) submatrices (minors). While conceptually straightforward, it becomes computationally very expensive for large n, with complexity growing factorially (O(n!)).
Row reduction (Gaussian elimination) is generally more efficient for larger matrices. By applying row operations that have known effects on the determinant (swapping rows multiplies by -1, scaling a row scales the determinant, adding a multiple of one row to another leaves it unchanged), the matrix is transformed into row echelon form (typically upper triangular). The determinant is then the product of the diagonal entries, adjusted by factors accumulated from row swaps and scaling. This method has a complexity closer to O(n³), making it practical for much larger matrices encountered in computations.
These courses cover various computational techniques for finding determinants.
Relationship to Eigenvalues and Matrix Invertibility
Determinants are deeply connected to two other critical matrix concepts: invertibility and eigenvalues. As mentioned earlier, a square matrix A is invertible if and only if det(A) ≠ 0. This is arguably the most fundamental application of the determinant concept in basic linear algebra, directly impacting the solvability of linear systems.
The connection to eigenvalues is also profound. An eigenvalue λ of a matrix A is a scalar such that Av = λv for some non-zero vector v (the eigenvector). This equation can be rewritten as (A - λI)v = 0, where I is the identity matrix. This means the matrix (A - λI) maps a non-zero vector v to the zero vector, implying that (A - λI) is not invertible. Consequently, its determinant must be zero: det(A - λI) = 0.
This equation, det(A - λI) = 0, is known as the characteristic equation of the matrix A. It's a polynomial equation in λ, and its roots are precisely the eigenvalues of A. Thus, finding eigenvalues boils down to finding the roots of a polynomial defined by a determinant. Furthermore, the determinant of a matrix is equal to the product of its eigenvalues (counting multiplicity), linking these concepts tightly.
These courses explore the connections between determinants, eigenvalues, and matrix properties like invertibility.
Determinants in Practical Applications
While determinants are a theoretical cornerstone of linear algebra, their practical utility extends across numerous scientific, engineering, and economic disciplines. They often appear implicitly within algorithms or as part of larger mathematical models used to describe and analyze real-world phenomena.
Engineering Systems Analysis
In various branches of engineering, particularly control systems and structural analysis, determinants play a vital role. In control theory, the stability of a linear system is often analyzed using the characteristic equation of the system's state-space matrix. The roots of this equation (eigenvalues), found by setting a determinant to zero, dictate whether the system is stable, unstable, or marginally stable.
In structural engineering, finite element analysis often involves solving large systems of linear equations representing the stresses and strains within a structure. The determinant of the stiffness matrix provides information about the structural integrity and solvability of the system. A zero or near-zero determinant might indicate instability or singularity issues in the model.
Electrical engineering also uses determinants in circuit analysis, particularly when dealing with systems of equations derived from Kirchhoff's laws or mesh/nodal analysis, especially in the context of AC circuits using complex impedance.
This course targets engineers and covers relevant matrix algebra, including determinants.
Economic Modeling
Economics, particularly macroeconomics and econometrics, frequently employs matrix algebra. Input-output models, developed by Wassily Leontief, use matrices to represent the interdependencies between different sectors of an economy. Solving these models often involves matrix inversion, where the determinant plays its role as a condition for solvability.
In econometrics, determinants appear in the context of multivariate statistical analysis. For example, in testing hypotheses involving multiple parameters (e.g., using likelihood ratio tests), test statistics are sometimes calculated using determinants of variance-covariance matrices. The Jacobian determinant also arises when transforming random variables in multivariate distributions.
Optimization problems in economics, solved using techniques like Lagrange multipliers, can lead to systems of equations whose solvability conditions involve determinants (specifically, determinants of bordered Hessians to check second-order conditions for constrained optima).
Computer Graphics and Geometry
In computer graphics, linear transformations (scaling, rotation, shearing) are fundamental operations represented by matrices. The determinant of a transformation matrix reveals how the transformation affects area or volume. A positive determinant indicates orientation is preserved, while a negative determinant indicates a reflection (orientation reversal). A determinant of 1 signifies a volume-preserving transformation (like rotation).
Determinants are also used in computational geometry algorithms. For instance, determining whether a point lies to the left or right of a directed line segment (or on the line itself) can be efficiently calculated using the sign of a 2x2 determinant involving the coordinates of the points. This is crucial for algorithms involving polygon orientation, convex hulls, and intersection tests.
Calculating the area of a triangle given its vertex coordinates, or the volume of a tetrahedron, can also be done directly using determinants, leveraging their geometric interpretation as scaling factors for area/volume.
Machine Learning and Statistics
Determinants find applications in machine learning and statistics, often related to multivariate distributions and matrix decompositions. The determinant of a covariance matrix, for example, is related to the volume of the data cloud in multivariate analysis. It appears in the probability density function of the multivariate normal distribution.
In techniques like Principal Component Analysis (PCA), although the core involves eigenvalue decomposition, understanding the properties of the covariance matrix (including its determinant) provides context. Some algorithms in probabilistic modeling or Bayesian inference might involve calculating determinants of matrices, particularly when dealing with Gaussian processes or evaluating likelihood functions.
Furthermore, understanding matrix invertibility (tied to non-zero determinants) is crucial when solving linear regression problems using the normal equations (XᵀX)β = Xᵀy, as the matrix XᵀX must be invertible.
These courses connect linear algebra, including determinants, to data science and machine learning.
These careers heavily utilize concepts from linear algebra and statistics.
Physics and Quantum Mechanics
Determinants appear in various areas of physics. In classical mechanics, the Jacobian determinant is used when changing coordinate systems (e.g., from Cartesian to polar or spherical coordinates) in multiple integrals. It represents the local scaling factor for volume elements under the coordinate transformation.
In quantum mechanics, determinants arise notably in the context of fermionic systems due to the Pauli exclusion principle. The wavefunction for a system of N identical fermions must be antisymmetric under the exchange of any two particles. This property is naturally satisfied by constructing the wavefunction as a Slater determinant, which is a determinant formed from single-particle wavefunctions.
Matrix mechanics, one of the formulations of quantum mechanics, heavily relies on linear algebra. While operators are often infinite-dimensional, finite-dimensional approximations and specific problems involve matrix representations where concepts like determinants and eigenvalues are applicable.
This book explores matrix applications, relevant to various scientific fields.
Formal Education Pathways
High School Preparation
While determinants themselves are typically introduced in advanced high school math courses or early university studies, a strong foundation laid in earlier high school mathematics is essential. Proficiency in algebra, including manipulating expressions, solving equations, and understanding functions, is fundamental. Familiarity with coordinate geometry also provides a helpful visual intuition for the transformations that matrices represent.
Courses covering basic matrix operations (addition, multiplication) and solving systems of linear equations using methods like substitution or elimination provide direct preparation. Exposure to polynomial equations is also beneficial, as finding eigenvalues involves solving the characteristic polynomial.
Students aiming for STEM fields where linear algebra is crucial should focus on developing strong analytical reasoning and problem-solving skills throughout their high school math curriculum. Taking the most rigorous math courses available, such as pre-calculus and potentially AP Calculus or introductory linear algebra if offered, is advisable.
These courses are suitable for advanced high school students or those reviewing foundational concepts before university.
Undergraduate Curriculum Integration
Determinants are a standard topic in introductory undergraduate linear algebra courses, typically taken by students in mathematics, computer science, engineering, physics, economics, and other quantitative fields. These courses usually cover the definition, properties, methods of computation (cofactor expansion, row reduction), and key applications like checking invertibility, solving systems (Cramer's Rule), and finding eigenvalues.
Beyond the initial introduction, determinants reappear in various contexts. In multivariable calculus, the Jacobian determinant is crucial for performing change of variables in multiple integrals. In differential equations courses, determinants are used to find eigenvalues for solving systems of linear differential equations and analyzing stability.
Numerical analysis courses might discuss the computational challenges and stability issues related to determinant calculation for large or ill-conditioned matrices. Abstract algebra courses may present determinants from a more theoretical perspective, exploring their properties within the framework of multilinear algebra.
These books are common texts or supplementary resources for undergraduate linear algebra.
Understanding multivariable calculus is often helpful alongside linear algebra.
Graduate-Level Specialization Options
At the graduate level, determinants are usually assumed knowledge, but they continue to appear in more advanced contexts. In functional analysis, the concept extends to Fredholm determinants for certain types of integral operators. In differential geometry and topology, determinants are related to volume forms and orientation.
Numerical linear algebra focuses on developing robust and efficient algorithms for matrix computations, including specialized techniques that might involve or relate to determinants for specific matrix structures (e.g., sparse matrices, structured matrices). Matrix theory courses delve deeper into advanced topics like tensor products and multilinear algebra, where determinants arise as specific instances of more general constructions.
Research in areas like random matrix theory, often applied in physics, statistics, and number theory, heavily involves analyzing the properties of determinants and eigenvalues of matrices with random entries. Theoretical computer science also encounters determinants in areas like algebraic complexity theory.
These more advanced books cover topics often encountered in graduate studies.
Research Frontiers
While the core theory of determinants for finite matrices is well-established, research involving determinants continues in various specialized areas. Algebraic complexity theory investigates the minimum number of operations required to compute determinants and related functions, with implications for the fundamental limits of computation.
Random matrix theory remains an active field, studying the statistical properties of determinants and eigenvalues of large random matrices, with applications ranging from nuclear physics to wireless communications and financial modeling. Connections between determinants and other areas of mathematics, such as number theory (e.g., via L-functions) and combinatorics (e.g., determinants counting specific graph structures), continue to be explored.
The development of quantum algorithms and the challenges of high-dimensional data analysis also present new contexts where determinant-related concepts might find novel applications or require new theoretical developments, particularly concerning computational efficiency and interpretation in very large-scale problems.
Online Learning Strategies
Effective Self-Study Methodologies
Learning about determinants effectively through self-study, especially using online resources, requires structure and discipline. Start by identifying a core learning resource, such as a comprehensive online course or a well-regarded textbook. Supplement this with other materials like video lectures, interactive applets demonstrating geometric interpretations, and practice problem sets.
A key strategy is active learning. Don't just passively watch videos or read text. Work through examples step-by-step, pause frequently to test your understanding, and attempt practice problems before looking at solutions. Focus on grasping the underlying concepts and geometric intuition, not just memorizing formulas or computational procedures.
Create a study schedule and stick to it. Break down the topic into smaller, manageable chunks (e.g., 2x2 determinants, properties, cofactor expansion, row reduction, eigenvalues). Regularly review previous material to reinforce your understanding. Using tools like OpenCourser to find and compare courses, and potentially save them to a personalized list using the "Save to list" feature, can help organize your learning path.
These courses offer structured paths for learning linear algebra concepts online.
Project-Based Learning Approaches
Applying knowledge through projects is an excellent way to solidify understanding and gain practical skills. Once you grasp the basics of determinants and matrix operations, consider projects like implementing determinant calculation algorithms (e.g., cofactor expansion, row reduction) in a programming language like Python (using libraries like NumPy for comparison, but coding the logic yourself initially).
Explore geometric applications: write code to visualize how 2x2 or 3x3 matrices transform shapes (like a unit square or cube) and how the determinant relates to the change in area or volume. Implement Cramer's rule to solve small systems of equations and compare its performance to other methods like Gaussian elimination.
For more advanced learners, projects could involve implementing eigenvalue/eigenvector calculations (which rely on determinants) or exploring applications in a specific domain, such as analyzing the stability of a simple control system model or performing basic image transformations using matrices.
Supplementing Formal Education
Online resources can significantly enhance formal education in mathematics. If you're struggling with determinants in a university course, online lectures or tutorials might offer alternative explanations or visualizations that click better with your learning style. Platforms often feature instructors with different teaching approaches, providing fresh perspectives.
Use online practice problem sets and quizzes for extra drilling and self-assessment beyond assigned homework. Interactive simulations can provide valuable intuition, especially for the geometric meaning of determinants. If your course moves quickly, online courses can allow you to revisit prerequisite topics or explore related concepts at your own pace.
Consider using online courses to get a head start before a formal course begins or to delve deeper into specific applications (like determinants in machine learning or engineering) that might only be touched upon briefly in a standard curriculum. The OpenCourser Learner's Guide offers tips on integrating online learning with traditional studies.
Building Practical Applications
Transitioning from theoretical understanding to practical application is crucial for career relevance. Focus on understanding why and where determinants are used in specific fields. If interested in data science, explore how covariance matrices and their determinants are used in multivariate statistics and algorithms like Gaussian Mixture Models.
If aiming for engineering, study examples of stability analysis in control systems or structural analysis problems where determinants arise. For computer graphics, work through examples of geometric transformations and collision detection algorithms that utilize determinant calculations.
Building a portfolio of small projects demonstrating these applications can be valuable for career development. Even simple implementations show initiative and practical understanding beyond textbook knowledge. Connect with online communities or forums focused on specific application domains (e.g., machine learning, game development) to see how these concepts are used in practice and potentially contribute to open-source projects.
This book focuses on the application side of linear algebra.
Career Progression with Determinants
Entry-Level Roles
Direct knowledge of determinant calculation might not be a daily task in many entry-level roles, but the underlying concepts of linear algebra are fundamental in numerous quantitative fields. Roles in data analysis, software development (especially in scientific computing, graphics, or simulation), quantitative analysis (finance), engineering (control systems, structural, electrical), and actuarial science often require a solid understanding of matrix operations, vector spaces, and solving linear systems – areas where determinants provide crucial theoretical underpinnings.
Entry-level positions often test foundational mathematical knowledge during interviews. Demonstrating a clear grasp of linear algebra concepts, including the significance and properties of determinants (like their relation to invertibility and eigenvalues), signals strong analytical aptitude valued by employers in technical fields.
Therefore, while "Determinant Expert" isn't a job title, proficiency in the linear algebra toolkit, including determinants, is often a prerequisite or a strong asset for securing entry-level positions in these domains.
Mid-Career Specialization
As professionals advance, specialization often involves deeper application of foundational knowledge. An engineer might specialize in advanced simulation or control system design, where sophisticated analysis relies heavily on eigenvalue problems (derived from determinants). A data scientist might move into developing novel machine learning algorithms or complex statistical models where understanding covariance matrices, Gaussian processes, or optimization techniques requires intimate knowledge of linear algebra.
A quantitative analyst ("quant") in finance might develop pricing models for complex derivatives or risk management systems that involve stochastic calculus and numerical methods rooted in linear algebra. Software engineers working on high-performance computing libraries, physics engines for games, or computer vision algorithms will frequently encounter and implement matrix-based algorithms where efficiency and numerical stability (related to properties like matrix condition numbers, which are connected to determinants and eigenvalues) are critical.
Mid-career roles often require not just applying standard techniques but adapting or developing new ones, demanding a robust theoretical understanding that includes the role and implications of determinants within broader mathematical frameworks.
Careers where linear algebra proficiency, including determinants, is valuable include:
Leadership Positions
In technical leadership positions (e.g., Principal Engineer, Research Scientist, Technical Lead, Quantitative Portfolio Manager), a deep understanding of fundamental principles like those in linear algebra remains crucial. Leaders often guide architectural decisions, select appropriate modeling techniques, troubleshoot complex problems, and mentor junior team members. This requires a holistic view of the mathematical and computational tools being used.
While day-to-day coding or calculation might decrease, the ability to reason abstractly about system behavior, model limitations, and potential numerical issues is paramount. Understanding concepts like matrix conditioning, stability, and the geometric interpretation of linear transformations (informed by determinants and eigenvalues) allows leaders to make sound technical judgments and guide research or development directions effectively.
Furthermore, communicating complex technical ideas to diverse audiences (including less technical stakeholders) requires clarity rooted in a firm grasp of the fundamentals. Explaining why a particular approach is chosen or what the limitations of a model are often traces back to core mathematical principles.
Cross-Industry Transferable Skills
The core skills developed while learning determinants – analytical reasoning, abstract thinking, problem-solving, understanding complex systems through mathematical models – are highly transferable across industries. Linear algebra provides a common language for describing problems in fields as diverse as finance, robotics, bioinformatics, logistics, and data science.
Mastery of concepts like matrix invertibility, eigenvalues, and the geometric meaning of determinants equips individuals with versatile tools for analyzing data, modeling systems, and developing algorithms. This mathematical maturity is valuable even if the specific application domain changes.
Therefore, investing time in understanding determinants and broader linear algebra concepts provides a durable skill set applicable to a wide range of quantitative and technical careers, facilitating mobility between different industries or roles that rely on mathematical modeling and computation. The U.S. Bureau of Labor Statistics highlights strong projected growth for mathematicians and statisticians, roles heavily reliant on these foundational skills.
Challenges in Determinant Applications
Computational Complexity
While determinants are theoretically elegant, their direct computation can be challenging for large matrices. Methods based on the definition, like cofactor expansion, have factorial time complexity (O(n!)), making them computationally infeasible for anything beyond very small matrices (n > 10-15 quickly becomes intractable).
Using row reduction (Gaussian elimination) is much more efficient, typically O(n³), but this can still be computationally intensive for the extremely large matrices encountered in modern data science, scientific computing, or large-scale simulations (where n can be in the millions). Developing algorithms that avoid direct determinant calculation or exploit specific matrix structures (like sparsity) is often necessary.
Consequently, while determinants provide crucial theoretical insights (like conditions for invertibility), direct computation is often bypassed in practical algorithms in favor of methods that achieve the same goal more efficiently (e.g., using LU decomposition to solve systems or check invertibility, or iterative methods to find eigenvalues).
Numerical Stability Issues
Beyond computational cost, calculating determinants can suffer from numerical instability, especially when using floating-point arithmetic on computers. Matrices that are "ill-conditioned" (close to being singular, meaning their determinant is close to zero) are particularly problematic. Small errors in the input matrix elements or rounding errors during computation can lead to large errors in the calculated determinant.
Checking if a determinant is exactly zero using floating-point numbers is unreliable. Instead, numerical analysts often work with concepts like the condition number of a matrix, which provides a more robust measure of how sensitive the matrix (and solutions involving it) is to perturbations. Algorithms are designed to be numerically stable, minimizing the accumulation and amplification of errors.
This means that relying solely on a computed determinant value, especially if it's close to zero, can be misleading in practice. Robust numerical methods often use alternative approaches to assess properties like invertibility or rank.
Interpretation Challenges in High Dimensions
The geometric interpretation of the determinant as a volume scaling factor is intuitive in 2D (area) and 3D (volume). However, this intuition becomes harder to grasp in high-dimensional spaces encountered in machine learning or complex system modeling. What does the "hypervolume" scaling factor represented by the determinant of a 1000x1000 matrix truly signify in a practical sense?
While mathematically sound, interpreting the magnitude of a determinant in very high dimensions requires careful consideration. Often, related concepts like eigenvalues (which describe scaling along specific directions) or the overall conditioning of the matrix provide more practical insights into the behavior of the transformation or the stability of the system being modeled.
The determinant might still be used formally (e.g., in the formula for a multivariate Gaussian), but its direct numerical value might be less informative than other matrix properties in high-dimensional settings.
Ethical Considerations
While determinants themselves are abstract mathematical objects, their application within larger systems, particularly in predictive modeling, data analysis, and automated decision-making, can intersect with ethical considerations. If determinants are part of algorithms used for credit scoring, hiring, or resource allocation, biases present in the input data or model structure can lead to unfair or discriminatory outcomes.
For example, if a model relies on matrix inversion (implicitly checking if a determinant is non-zero) based on potentially biased data, the resulting decisions could perpetuate societal inequalities. Understanding the limitations and potential failure modes of mathematical models, including issues related to matrix conditioning and numerical stability which are linked to determinants, is crucial for responsible development and deployment.
Practitioners must be aware of how the mathematical tools they use function within the broader context of their application and consider the potential societal impacts, ensuring models are fair, transparent, and robust.
Future of Determinant Theory
Quantum Computing Implications
Quantum computing presents both challenges and opportunities related to linear algebra computations. While Shor's algorithm famously provides exponential speedup for factoring (not directly determinant-related), the HHL algorithm (Harrow, Hassidim, Lloyd) offers potential exponential speedup for solving certain systems of linear equations under specific conditions. This implicitly relates to matrix inversion and thus determinants.
However, the practical realization of these speedups faces significant hurdles in terms of quantum hardware development and error correction. Furthermore, quantum algorithms often provide probabilistic outputs or specific information about the solution (like expectation values) rather than the full classical solution vector or the exact determinant value.
Research continues into developing quantum algorithms for other linear algebra tasks, potentially including more direct determinant estimation or eigenvalue problems. The impact of quantum computing on classical numerical linear algebra, including determinant calculations, is an active area of investigation, but widespread practical advantages for these specific tasks are likely still some way off.
High-Dimensional Data Challenges
The increasing prevalence of high-dimensional data ("big data") poses challenges for many classical methods, including those involving determinants. As mentioned, direct determinant computation becomes expensive, and interpretation becomes less intuitive in high dimensions. Moreover, phenomena like the "curse of dimensionality" can affect the behavior of geometric concepts and statistical methods.
Research focuses on developing techniques suitable for high-dimensional settings. This includes random matrix theory insights, dimensionality reduction techniques (like PCA, which involves eigenvalues derived from determinants), and algorithms that exploit sparsity or other structures often present in real-world high-dimensional data. Methods that approximate determinants or focus on related quantities (like the log-determinant, often used in optimization) might be preferred.
The theoretical role of determinants remains, but practical computation and application in the face of massive datasets require ongoing innovation in algorithms and numerical methods.
Interdisciplinary Research Opportunities
Determinants and linear algebra continue to find new applications across disciplines. Fields like network science (analyzing graph Laplacians, where determinants relate to spanning trees), systems biology (modeling complex biological networks), materials science (analyzing material properties via tensor representations), and computational social science rely heavily on matrix methods.
The interplay between linear algebra and machine learning is particularly fertile ground. Understanding the geometry of high-dimensional data spaces, developing new optimization algorithms, and analyzing the properties of neural network weight matrices often involves sophisticated linear algebraic tools where determinants and related concepts (eigenvalues, singular values) play a role.
Opportunities exist for mathematicians, computer scientists, and domain experts to collaborate on developing and applying advanced linear algebraic techniques, including those related to determinants, to solve pressing problems in diverse scientific and technological areas. Resources like university research pages often showcase such interdisciplinary work (e.g., exploring applications at institutions like MIT).
Automation Impacts on Determinant Calculations
Software libraries (like NumPy/SciPy in Python, MATLAB, R's base functions) have largely automated the computation of determinants and related matrix operations (inversion, eigenvalue calculation). Few practitioners manually calculate determinants for anything larger than 3x3 matrices today; they rely on highly optimized and numerically robust library functions.
This automation shifts the emphasis from computational skill to conceptual understanding. The value lies not in being able to compute a determinant by hand, but in understanding what it represents, when it is relevant, what its properties imply, and how to interpret the results provided by software tools, including potential numerical issues.
Future developments in AI and automated theorem proving might further automate aspects of symbolic manipulation or even derivation of properties related to determinants. However, the need for human understanding of the underlying mathematical principles to guide problem formulation, model selection, and result interpretation will remain essential.
Frequently Asked Questions
Is determinant knowledge essential for data science careers?
While directly calculating determinants might be infrequent, a solid understanding of the underlying linear algebra concepts they represent (invertibility, eigenvalues, geometric transformations) is crucial for data science. Many machine learning algorithms (PCA, LDA, Gaussian models, regression) are built upon linear algebra. Understanding determinants helps grasp why these algorithms work, their limitations, and how to interpret their results. So, while not always explicitly used daily, the conceptual knowledge is foundational.
How does AI automation affect determinant-related jobs?
AI and automation primarily affect the computational aspect. Software readily calculates determinants. This doesn't eliminate the need for understanding them; rather, it shifts the focus. Professionals need to understand the theory to choose appropriate methods, interpret automated outputs correctly (including potential numerical errors), and apply the concepts within larger problem-solving contexts. Jobs requiring rote calculation may decrease, but those requiring conceptual understanding, modeling, and interpretation remain valuable.
What industries value determinant expertise most?
Expertise related to determinants (as part of broader linear algebra knowledge) is valued in industries relying heavily on mathematical modeling, simulation, and data analysis. This includes aerospace, automotive, and mechanical engineering (for structural analysis, dynamics, control systems), computer graphics and game development, finance (quantitative analysis, risk management), data science and machine learning, scientific research (physics, economics, statistics), and operations research.
Can I specialize in determinants without a PhD?
It's unlikely one would "specialize" solely in determinants outside of pure mathematics research (which typically requires a PhD). However, one can specialize in fields where advanced linear algebra, including a deep understanding of determinants, eigenvalues, and matrix decompositions, is critical. This could be in numerical analysis, scientific computing, control theory, quantum computing algorithm development, or theoretical machine learning, often pursued at the Master's or PhD level, or through significant industry experience and self-study.
How transferable are determinant skills between industries?
The core mathematical understanding associated with determinants – linear transformations, system solvability, eigenvalues, geometric intuition – is highly transferable. Linear algebra is a universal language in quantitative fields. The ability to think abstractly, model systems mathematically, and understand matrix properties allows professionals to adapt their skills to different application domains, whether moving from engineering to data science or from finance to physics simulation.
What emerging fields use determinant theory?
Emerging fields continue to find uses for linear algebra, including determinant-related concepts. Quantum computing relies heavily on linear algebra for describing quantum states and operations. Advanced machine learning areas like geometric deep learning use algebraic and geometric concepts extensively. Fields like topological data analysis use algebraic tools to understand the shape of data. As computational power grows, complex simulations in materials science, climate modeling, and systems biology increasingly rely on sophisticated numerical linear algebra.
Topics related to linear algebra, like calculus, are often studied concurrently.
Differential equations are another related field where determinants play a role.
Understanding determinants is a gateway to the powerful world of linear algebra and its vast applications. Whether pursued through formal education or online learning via platforms like OpenCourser, mastering this topic builds a strong foundation for numerous rewarding technical and scientific careers.